Irrational Numbers

Background

You might have noticed, when talking about Rational Numbers, that when we dealt with decimals, we only dealt with those that either ended (the technical term is terminated), like 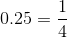, or repeated, like  . So what about decimals that neither terminate or repeat?

Question

What is an irrational number? How many of them sit between 0 and 1? 

Answer

The definition of an irrational number is one of exclusion (in other words, an irrational number is defined by what it isn't rather than what it is). An irrational number is neither rational (that is, can be made be made by a fraction of integers with the denominator being non-zero) nor is it imaginary (we'll talk about what this is in a later entry).

There are an infinite number of irrational numbers between 0 and 1. 

Analysis

We alluded to what an irrational number is in the Background, but let's go over what it is and isn't in more detail here.

Let's take a look at 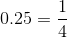, and specifically the decimal, 0.25. We can express this terminating decimal as a fraction:



And we can go through a process with a repeating decimal to turn it into a fraction:


   

we subtract them to get:





But with an irrational number, we can't simply put a terminated decimal over an appropriate power of 10 (like 100 which is what we used for 0.25) nor can we go through a process to subtract out the repeating decimal like we did with . An irrational number has a decimal that doesn't repeat or terminate, like:

0.123456789101112131415...

The decimals can be random, or there can be a pattern that doesn't repeat (like writing out the natural numbers in order like above).

Notice that we can replace the first 1 in the series with any of the infinite number of natural numbers, and that is simply the first digit (we could do that with any digit!). Therefore, we can say that there are an infinite number of irrational numbers between 0 and 1.

Vocabulary used:

For more information check out these links (comment to add your favourite link):

Where might you have come from?

Fact-orials Index

Numbers:

• Rational Numbers

Where might we go?

Numbers:

• Pi
• Real Numbers