Let's work some problems involving addition of rational numbers!
Question
Evaluate the following:
Answer
Analysis
Question 1
The first thing we need to do is get common denominators. I'll do that by first doing prime factorizations of the denominators:
6 = 2 x 3
3 = 3
10 = 2 x 5
Now we look at each prime number and grab the biggest group of each.
There's a single 2, a single 3, and a single 5, which means the lowest common denominator is:
2 x 3 x 5 = 30
Now let's get our fractions set up so we can do the math:



Now we want our answer in "lowest terms" - which means we're going to look if we can take out any forms of 1 that are lurking in that fraction. And there are:


Therefore:

Question 2
Let's put all of the term into improper fraction terms.
We have these terms to work with:

The first term is already in fraction form so we're set here.
For the second term, we multiply the whole number by the denominator, then add that to the numerator, like this:

For the third term, we go through the process of converting a repeating decimal to a fraction:

We've split out the 8 from the repeating decimal, so now we can focus on that decimal:


Therefore:

We combine the whole number with the fraction:

Ok - we can now rewrite our original question:

Now we need a common denominator. I'll do the prime factorizations of the denominators:
7 = 7
2 = 2
9 = 3 x 3
Ok - we need a single 2, two 3's, and a 7. That gives:
7 x 2 x 3 x 3 = 126
Let's get our fractions set up for the addition:


Let's work through to see if we need to reduce this fraction.
We know that 126 has prime factors 2, 3, and 7. If 1613 is divisible by any of these, then we'll have an opportunity to do some reducing.
Is 1613 divisible by 2? No - it's not even.
Is 1613 divisible by 3? No - the sum of its digits does not sum to a number divisible by 3.
Is 1613 divisible by 7? No - this one we can try on a calculator or we could work it through using the divisibility facts.
Therefore, the fraction can't be reduced and we can keep the answer as
 .
.So what is this number in mixed number form and as a decimal?
As a decimal, it works out to be 12.8015873016
As a mixed number, it works out to be

Therefore:

Vocabulary used:
For more information check out these links (comment to add your favourite link):
Where might you have come from?
Fact-orials Index
Operations with different kinds of numbers:
- Addition, Subtraction, and Integers - Practice Problems
- Addition, Subtraction, and Rational Numbers
- Multiplication, Division, and Rational Numbers - Practice Problems
Relations:
Where might we go?
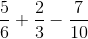



No comments:
Post a Comment
Hi there - I'm glad to see you are thinking about or maybe even getting ready to post a comment! I moderate all comments so please be patient while I hit the "ok" button on yours. Feel free to make suggestions on web resources to add, directions the entries should go,... whatever. And thanks again for leaving some feedback!